Conference paper on micropolar coupling method accepted for ECCOMAS Congress 2016.

A SEMI-IMPLICIT MICROPLAR DISCRETE-TO-CONTINUUM METHOD FOR GRANULAR MATERIALS

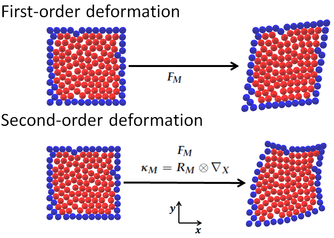
Kun Wang, WaiChing Sun

Department of Civil Engineering and Engineering Mechanics, Columbia University
614 SW Mudd, Mail Code: 4709, New York, NY 10027
e-mail: {kw2534,wsun}@columbia.edu

Keywords: Micropolar Continua, Discrete-to-Continuum, Granular Matters, Length Scale, Strain Localization.

Abstract. A micropolar discrete-continuum coupling model is proposed to link the collectively particulate mechanical simulations at high-order representative elementary volume to field-scale boundary value problems. By incorporating high-order kinematics to the homogenization procedure, contact moment and force exerted on grain contacts are homogenized into a non-symmetric Cauchy stress and higher-order couple stress. These stress measures in return become the constitutive updates for the macroscopic finite element model for micropolar continua. Unlike the non-lcoal weighted averaging models in which the intrinsic length scale must be a prior knowledge to compute the nonlocal damage or strain measures, the proposed model introduces the physical length scale directly through the higher-order kinematics. As a result, there is no need to tune or adjust the intrinsic length scale. Furthermore, since constitutive updates are provided directly from micro-structures, there is also no need to calibrate any high-order material parameters that are difficult to infer from experiments. These salient features are demonstrated by numerical examples. The classical result from Mindlin is used as a benchmark to verify the proposed model.